Testing of power transformer – Measurement of impedance voltage and load loss

 

 

Purpose of the measurement

The measurement is carried out to determine the load-losses of the transformer and the impedance voltage at rated frequency and rated current.

 The measurements are made separately for each winding pair (e.g., the pairs 1-2, 1-3 and 2-3 for a three-winding transformer), and furthermore on the principal and extreme tappings.

 

Apparatus and measuring circuit

On Figure 1 above (Circuit for the impedance and load-loss measurement) there are following figures:

• G₁ – Supply generator
• T₁ – Step-up transformer
• T₂ – Transformer to be tested
• T₃ – Current transformers
• T₄ – Voltage transformers
• P₁ – Wattmeters
• P₂ – Ammeters (r.m.s. value)
• P₃ – Voltmeters (r.m.s. value)
• C₁ – Capacitor bank

The supply and measuring facilities are not described here. Current is generally supplied to the h.v. winding and the l.v. winding is short-circuited.

Performance of the measurement

If the reactive power supplied by the generator G₁ is not sufficient when measuring large transformers, a capacitor bank C₁ is used to compensate part of the inductive reactive power taken by the transformer T₂.The voltage of the supply generator is raised until the current has attained the required value (25…100 % of the rated current according to the standard 4.1).

In order to increase the accuracy of readings will be taken at several current values near the required level. If a winding in the pair to be measured is equipped with an off-circuit or on-load tap-changer. the measurements are carried out on the principal and extreme tappings.

 The readings have to be taken as quickly as possible, because the windings tend to warm up due to the current and the loss values obtained in the measurement are accordingly too high.

It the transformer has more than two windings all winding pairs are measured separately.

Results

Corrections caused by the instrument transformers are made to the measured current, voltage and power values. The power value correction caused by the phase displacement is calculated as follows:

Equation 4.1

Where:

• P_c = corrected power
• P_e = power read from the meters
• δ_u = phase displacement of the voltage transformer in minutes
• δ_i = phase displacement of the current transformer in minutes
• ϕ = phase angle between current and voltage in the measurement (ϕ is positive at inductive load)
• K = correction

The correction K obtained from equation 4.1 is shown as a set of curves in Figure 4.2.
The corrections caused by the instrument transformers are made separately for each phase, because different phases may have different power factors and the phase displacements of the instrument transformers are generally different.
If the measuring current I_m deviates from the rated current I_N, the power P_km and the voltage U_km at rated current are obtained by applying corrections to the values P_c and U_c relating to the measuring current.
The corrections are made as follows:

Equation 4.2

Equation 4.3

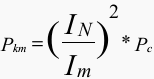
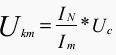
The correction caused by the phase displacement of instrument transformers (Figure 2):

 

Where:

• K – correction in percent,
• δ_u – δ_i – phase displacement in minutes
• cosδ – power factor of the measurement.

The sign of K is the same as that of δ_u – δ_i.
Mean values are calculated of the values corrected to the rated current and the mean values are used in the following. According to the standards the measured value of the losses shall be corrected to a winding temperature of 75° C (80° C, if the oil circulation is forced and directed).
The transformer is at ambient temperature when the measurements are carried out. and the loss values are corrected to the reference temperature 75° C according to the standards as follows.
The d.c. losses P_Om at the measuring temperature ϑ_m are calculated using the resistance values R_1m and R_2m obtained in the resistance measurement (for windings 1 and 2 between line terminals):

Equation 4.4

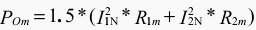
The additional losses Pamat the measuring temperature are:

Equation 4.5

Here P_km is the measured power, to which the corrections caused by the instrument transformer have been made, and which is corrected to the rated current according to equation (4.2).
The short-circuit impedance Z_km and resistance R_km at the measureing temperature are:

Equation 4.6

Equation 4.7

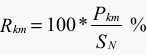
• U_km is the measured short-circuit voltage corrected according to Equation (4.3);
• U_N is the rated voltage and
• S_N is the rated power.

The short circuit reactance X_k does not depend on the losses and X_k is the same at the measuring temperature (ϑ_m) and the reference temperature (75 °C), hence:

Equation 4.8

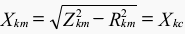
When the losses are corrected to 75° C, it is assumed that d.c. losses vary directly with resistance and the additional losses inversely with resistance. The losses corrected to 75° C are obtained as follows:

Equation 4.9

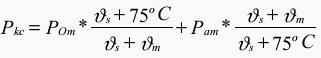
Where:
ϑs = 235° C for Copper
ϑs = 225° C for Aluminium
Now the short circuit resistance R_kc and the short circuit impedance Z_kc at the reference temperature can be determined:

Equation 4.10

 

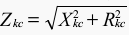    Equation 4.11

Results

The report indicates  for each winding pair the power S_N and the following values corrected to 75° C and relating to the principal and extreme tappings.

• D.C. losses P_Oc (PDC)
• Additional losses P_ac (PA)
• Load losses P_kc (PK)
• Short circuit resistance R_kc (RK)
• Short circuit reaactance X_kc (XK)
• Short circuit impedance Z_kc (ZK)

 

Read More

2. No-Load Excitation Current

This current is measured in the winding used to excite the transformer with the other windings open-circuited. It is generally expressed in percent of the rated current of the winding. No-load excitation current is not sinusoidal and contains, as we have seen, odd harmonics (predominantly third harmonic current).

The ammeter used to record the no-load excitation current is an RMS meter which reads the square root of the sum of the squares of the harmonic currents.

Read More

Transformer Routine Test – Measurement Of No-Load Loss And Current

Introduction to test

The no-load losses are very much related to the operational performance of a transformer. As long as the transformer is operated, these losses occur. For this reason, no load losses are very important for operational economy. No-load losses are also used in the heating test.
The no-load loss and current measurements of a transformer are made while one of the windings (usually the HV winding) is kept open and the other winding is supplied at the rated voltage and frequency.

 

During this test the no-load current (Io) and the no-load losses (Po) are measured.

The measured losses depend heavily on the applied voltage waveform and frequency. For this reason, the waveform of the voltage should be very sinusoidal and at rated frequency.
Normally, the measurements are made while the supply voltage is increased at equal intervals from 90% to 115% of the transformer rated voltage (Un) and this way the values at the rated voltage can also be found.

No-load losses and currents

The no-load losses of a transformer are grouped in three main topics:

1. Iron losses at the core of the transformer,
2. Dielectric losses at the insulating material and
3. The copper losses due to no-load current.

The last two of them are very small in value and can be ignored.
So, only the iron losses are considered in determining the no-load losses.

Measuring circuit and performing the measurement

In general according to the standards, if there is less than 3% difference between the effective (U) value and the average (U’) value of the supply voltage, the shape of the wave is considered as appropriate for measurements.

If the supply voltage is different than sinusoid, the measured no-load losses have to be corrected by a calculation. In this case, the effective (r.m.s.) value and the average (mean) value of the voltage are different. If the readings of both voltmeter are equal, there is no need for correction.

During measurements, the supply voltage U´ is supplied to the transformer by the average value voltmeter. In this way, the foreseen induction is formed and as a result of this, the hysteresis losses are measured correctly. The eddy-current losses should be corrected according to equation below.
P_m = P₀ · (P₁ + k · P₂)
P_m: Measured loss
P₀: No-load losses where the voltage is sinusoidal
Here: P₀ = P_h + P_E = k₁ · f + k₂ · f²
k = [ U / U’ ]²
P₁: The hysteresis loss ratio in total losses (P_h) = k₁ · f
P₂: The eddy-curent loss ratio in total losses (P_E) = k₂ · f²
At 50 Hz and 60 Hz, in cold oriented sheet steel, P₁ = P₂ = % 50. So, the P₀ no-load loss becomes:
P_o = P_m / (P₁ + k · P₂)   where P₁ = P₂ = 0,5
According to IEC 60076-1: P_m = P₀ · (1 + d)   where d = [ (U’ – U) / U’ ]

During no-load loss measurement, the effective value of the no-load current of the transformer is measured as well. In general, in three phase transformers, evaluation is made according to the average of the three phase currents.

Before the no-load measurements, the transformer might have been magnetised by direct current and it’s components (resistance measurement or impulse tests).
For this reason, the core has to be demagnetised. To do this, it has to be supplied by a voltage value (increasing and decreasing between the maximum and minimum voltage values for a few minutes) higher than the rated voltage for a certain time and then the measurements can be made.
The no-load currents are neither symmetrical nor of equal amplitude in three phase transformers. The phase angles between voltages and currents may be different for each of three phases.
For this reason, the wattmeter readings on each of the three phases may not be equal. Sometimes one of the wattmeter values can be 0 (zero) or negative (-).

Read More